In the past year or two, a movement toward curriculum change in college-level music theory ("reform" is the word more often used) has rapidly accelerated. Research had already expanded notably into new repertoires, especially music of all styles in the late 20th and 21st centuries. The journal demonstrating this most strongly is Music Theory Online (link), a venerable peer-reviewed journal of the Society for Music Theory, and one can also see the effects on teaching in recent volumes of the Journal of Music Theory Pedagogy (link). Other major journals are beginning to follow their lead.
Reading recently about one group's proposal to remove or severely limit the use of chorale harmonizations (almost always those by J. S. Bach), I decided to experiment with another change. What if large-market music theory textbooks took the dominant ninth chord out of its late-chapter ghetto and put it at or near the beginning of the book instead?
I'll use the 8th edition of Kostka, Payne, and Almén, Tonal Harmony with an Introduction to Post-Tonal Music (2017; publisher link) as my representative example. The book has 28 chapters. The topics covered are: in the first four, fundamentals; in the next eleven, diatonic triads and seventh chords; in the following ten, chromaticism; and in the final three, post-tonal theory. The second section on chromaticism encompasses chapters 21-25: 21 & 22 introduce the usual Neapolitan chord and augmented sixths, which are already found in 17th century sources and become staples of expressive harmonic practices in the 18th century; 23 is on enharmonic spellings; 24 is titled "further elements of the harmonic vocabulary"; and 25 carries us through the late 19th century.
I am now switching editions because the publisher's link for the 8th edition, which I do not own, does not show chapter subheadings. Some time ago I purchased a copy of the 4th edition (2004) for $1.00 at our local Half-Price Books Outlet store. A comparison of the tables of contents for the 4th and 8th editions suggests little has changed overall. The "further elements" chapter has five sections, the first two being on altered dominants. In the third section we finally reach "ninth, eleventh, and thirteenth chords." There are five musical examples, two from Beethoven, two from Schumann, and one the inevitable Franck Violin Sonata opening. Only the latter shows the major dominant ninth; three are Vb9, and one is ii9.
From less than four pages at the back of the book to a place of honor at or near the beginning of the curriculum would seem an impossible task, but actually I found it easy--but only up to a point. The difficulty was repertoire examples. I'll report on that in a subsequent post.
To begin: I assume that the student has covered fundamentals, including clefs (at least treble and bass), note names, intervals, scales (at least major and minor), and spelling triads and seventh chords. Knowing something about Roman numeral chord labels and jazz/popular chord symbols would be helpful, though they could be taught in conjunction with the text here.
Here are the four types of triads: major, minor, diminished, and augmented. The first three can be found in the major scale. The first line of labels is from jazz/popular-music theory; since labels can vary quite a bit, I chose to use the ones on the Berklee College of Music page Glossary of Terms (link) under “Terms Used in Harmony [1-4].” The second line has labels associated with guitar chord symbols.
The third line has Roman numeral labels, the type most commonly used in traditional undergraduate harmony textbooks. An alternate version you’ll sometimes see doesn’t have distinctions of “chord quality” (that is, major, minor, diminished, augmented) but just capital letters, so for the first three in the graphic I, II, VII. This system has trouble with accidentals so there’s no easy label for the last one, and in general this “all-caps” scheme is less useful than the others, and worse, although it does simplify things, it forces one into accepting a model where everything else is a variant of the scale-based triad.
Seventh chords add a scale note a third above the triad. The major triads produce two very different types. The first one is the “major-minor seventh chord” (major triad plus a minor third) or “dominant seventh” or “V7” or just “seventh chord” or “7”—in most music of the last century when you say “seventh chord” this one is the default. Notice that the label in the graphic has no qualifier—it’s just “G7.”
The second chord in the graphic is the other one built on the major triad: the “major-major seventh chord” or “major seventh chord.” It requires a qualifier “Maj” or “M” except in Roman numerals, which, oddly, distinguish quality of triads but not seventh chords; in the graphic below, V7 and I7 look the same, but they’re not.
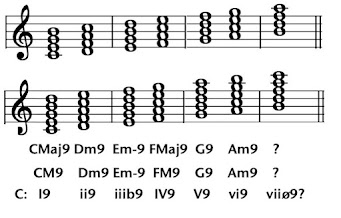
In the harmonic series, that wonderful source of symmetries and patterns in musical sounds, the dominant ninth shows up immediately after the dominant seventh. In the graphic below, first there is the G major triad (nos. 1-6), then the seventh (at position no. 7), then the ninth (at position no. 9; see the arrow!). That’s the explanation for this essay’s title, by the way: Harmony at the ninth. The red brackets show two ways to read the dominant ninth chord with consecutive tones of the harmonic series. The lower bracket hints at the traditional derivation of ninth chords by adding a third above the seventh.
We’ve reached the dominant ninth through chordal derivations so far, but there is another way to look at it, through the major scale. In this graphic, a C major scale appears at the left, my parsing of it at the right. Of the seven pitches, the dominant ninth (above) uses up five of them, whereas the C major triad (below) has only three. The practical implication of this—not lost on 20th century musicians especially—is that the dominant ninth can easily be used to harmonize quite a few melody tones.
In the common sequence of topics, this is where I would either keep on going and introduce the last two of the so-called “extended chords”—the eleventh and thirteenth chords—or I would discuss voice-leading for the dominant ninth chord. Although I acknowledge, just like I did with the ninth chords, that stacking up the thirds makes these chords easy to spell and remember, the problem is that the “extended chord” scheme is completely wrong historically. First of all, the eleventh and thirteenth chords both derive from substitution, not from adding more thirds: the eleventh takes the place of the tenth and the thirteenth takes the place of the twelfth. Second, one almost never sees these chords in their full form with six or seven notes: two or even three notes are commonly omitted. Discussing them now, in other words, would take us far afield from our main topic, which is how we can learn some essentials of tonal harmony by starting from the dominant ninth chord.
