Updated index

 I have published an updated index to this blog and essays about scale degree ^6 and the dominant ninth chord on the Texas ScholarWorks platform. Here is the link.

Rise and fall of the dominant ninth chord

A one-paragraph historical narrative: 

The major dominant-ninth chord* ("V9") gradually became a significant stylistic element in European and European-influenced music over the course of the nineteenth century. Early on it appeared in dance-based and song genres—notably, Schubert’s—and from there found its way onto the musical stage by the mid-1830s in both comic and dramatic works, eventually becoming associated with Wagnerian opera. By 1890, the two practices—exemplified by Wagner and Johann Strauss, jr.—were firmly established and can be found in a majority of the music from that point through the first half of the 20th century, including some concert music, but especially in musicals, salon or recital pieces, and commercial song repertoires. Before the end of the 19th century the major dominant-ninth chord had also established itself as one of the characteristic sounds of contemporary or Impressionist concert music, in part because of its close relation to the whole-tone scale (four notes out of five). Although that style did persist into the 1930s, already by 1925 the major dominant-ninth sound was considered passé and was often actively avoided.      (text edited 2024-09-23)

----

Find here an updated index of essays published on the Texas ScholarWorks platform, with abstracts and links: --> link. Essays on the dominant ninth are in §2, beginning on p. 10. Within this blog, of course, the search function can be used to locate specific names, titles, etc., and post titles can be browsed in the sidebar.

Altered dominants and the whole-tone scale

  In the entry for 28 October 2018, after explaining that the blog concerns only the major dominant-ninth chord--such as C: V9, G9, or A9, etc.--not what I call the minor dominant-ninth, or dominant 7th plus b9, or any of the non-dominant ninth chords, I wrote:

Still another exclusion from this blog is the dominant-ninth chord with altered fifth. These chords also begin to appear with some frequency in the 1890s. The version with raised fifth is more common; so, in C major G-B-D-F-A becomes G-B-D#-F-A, which happens to form a whole-tone scale pentachord also: D#-F-G-A-B [see the second example under (d) below]. Less common is the dominant ninth with lowered fifth, so: G-B-Db-F-A  [see the first example under (d) below]. This one, too, can be spelled in scalar form as a whole-tone pentachord: F-G-A-B-Db.

I'm going to take that back a bit, because the derivations of these chords are of historical interest for the familiar story of their connecting the dominant seventh and ninth with the whole-tone scale. Both appear in music first as alterations of V or of V7. 

The dominant seventh with lowered fifth was normally used in second inversion from the 1870s on. The first example under (e) below shows the dominant ninth and its alteration with the lowered fifth first and then the progression with V(b5)7 from which the latter is derived. Two points about it: exploited by Richard Strauss and others influenced by him, V(b5) is sometimes called the "Strauss chord" and normally appeared with the fifth in the bass; although the progression is functionally V(b9)–I, its notes are identical to IV: Fr+6-V. 

The chord with raised fifth, as V(#5)--see "from" in the second example under (e) below--already appears in galant-style music in the second half of the 18th century in the form V7(#5)/IV–IV; it has been associated particularly with Mozart, though he was by no means the only person to use it. The version with the ninth is unlikely before 1890.

Here are two simple (made-up) examples of scalar elaboration, showing the whole-tone scale as a melodic figure. At (f), G9 is first, but the scale is over V(b9) with the lowered fifth in the bass.  At (g), the fifth is missing in the G9; the raised fifth (D#) appears as leading into E6.

Symmetrical 5-chords

Last time I discussed symmetry and introduced the 7 (out of 38) 7-note sets/scales/chords that have it, besides the chromatic set and the major scale. Here are the corresponding 5-note sets, again in the interest of contextualizing the pitch content of 5-34, which is the major dominant ninth chord. 

All are "internally symmetrical" as defined in the previous post, and all are "completely symmetrical" by (1) some single note, or (2) the gap between two adjacent notes, as defined in the previous post. Though it's just another way of conceiving rule 2, a rule (3) makes a "double axis"--two notes at the center. Here are examples: type (1): 5-15 & 5-22; type (2): 5-34; type (3) 5-8.

To expand the contextualizing even more (and, granted, to wander a bit off-topic for this blog), here are some comments on these sets. Traits of most are clear, but a couple are more complex. The diminished triad frames 5-8 and 5Z12. 5Z17 as shown in the first example above is five notes of a C# minor scale (if C4 is B#3), 5-33 is most of the whole-tone scale, and 5-34 is the major dominant ninth. 5-15 has four notes of a whole-tone scale, here C4-D4-F#4-G#4 (if C is B#, this is the "French +6"), but also three chromatic notes and a quartal/quintal chord as G#-C#-F# or F#-C#-G#. 5-22 seems like a mash-up of three triads: C major, c# minor, and C+. And 5Z37 tucks a chromatic fragment D#-E-F into the middle of an augmented triad. The point of interest is that the properties of each of these sheds light on the 7-note complement. I'll explore that in the next post.

Here are two additional ways to think about the 5-note sets: as written, voiced chords, and in brief musical passages. First, the 5-8 below shows something not so obvious in the scalar version: this is a D9 with both 9 and b9.

Using the voicings of the chords above, here are short musical examples incorporating them (and where I can manage it, including V9 chords).

Symmetrical 5-chords and 7-chords

In an earlier "Curiosities" post (link), I discussed the symmetry of the major scale: starting on scale degree ^2, the inversion function, "I", produces all the same notes. Here is the first example again:

Only a small percentage of scales/pitch-sets/pc-sets in the 12-tone equal-tempered system are capable of this. Here are the best known. The chromatic scale is obvious; it has all 12 notes in a half-step sequence: of course, inversion will create the same ones. The whole-tone scale is pretty much the same, but with whole steps. The diminished scale is a little different: it's not symmetrical on a note but between notes. At (d) I have drawn a line between C4 and C#4 and then applied inversion from C4 down and C#4 up. In fact, the diminished scale is so structured that you can do this by drawing a line between any two adjacent notes of the scale.

Thus there are two sorts of scale or set symmetry: (1) from a note, as in the chromatic scale, major scale, and whole-tone scale; (2) from between notes, as in the diminished scale. (The latter can also be understood in terms of note pairs, or C4-C#4 together here.)

For a small number of sets/scales/chords, you can apply these within: I call this "internal symmetry," where you can generate a set/scale/chord from a sequence half its size. It's not common, either, but among the 5-note sets that have it is the major dominant ninth chord:

Btw, you can't find symmetry (1) with the entire V9 chord from its root--if you try it with the G9 above, that is, invert from G4 downward, you'll get an F9 instead, see (a) below. That's because the axis of symmetry is, you guessed it, scale degree ^2, just like the major scale figure at the top of this post--it's D5 at (b) below.

If we take a "scale-size chunk" of seven notes, in this case the pc complement of C: V9--that is, the seven notes in the chromatic scale that aren't in this V9--you can see that the resulting scale is also internally symmetrical. It also happens to be what is often called the "melodic minor scale," here C# minor.

Here are seven additional 7-note sets, beginning with two that have "type 1" symmetry like the major scale. These are followed by five with internal symmetry.

What this table does is to begin to put both 5-34, the pc set of the major dominant ninth, and 7-34, the ascending minor scale and the complement of V9, into a bit more general pitch-design context.

------

* The literature on scales and sets is understandably large. As I have explained a number of times, I am retired and don't feel the need to tot up the usual scholarly citations. I can say (a) that very little if anything I've said above is new, but that's not the point--it is to gather and discuss information in terms of the blog's topic; (b) if you want to explore more, the literature on set theory, pitch-class sets, and symmetry or symmetrical relations is the best place to go. Joe Straus's atonal theory textbook is the standard. Of online sites, I particularly like this one: "A Brief Introduction to Pitch-Class Set Analysis" (link) from Mount Alison University in Sackville, New Brunswick. It was made more than 20 years ago but is still available, informative, and easy to use.

Textbooks, update, part 6

My last post added to the series on traditional harmony textbooks (link to first post) discussion of a modern harmony text from the 1950s. Vincent Persichetti doesn't have anything negative to say specifically about the major dominant ninth chord, but as I wrote, he "greatly favors other seventh and ninth chord types over the major dominant ninth, which does appear in a reference example of the same kind of sequential progression that we saw in Mitchell and Ottman, but only once in the chapter's nine composed examples."

Just a few years earlier, Paul Hindemith indirectly offered an explanation: the major dominant ninth harmony (like its close cousin the half-diminished seventh) was too strongly associated with 19th century music. Hindemith was a student in the years before WWI, and matured as a musician and composer in the years immediately following, when the aesthetics and practices of the earlier era, especially anything Wagnerian, were soundly rejected. Thus there is a definite sarcasm in his proposing composition exercises for the harmonium, that "primitive wheezebox": "Is not treasuring such a pitiful instrument like preaching the virtue of poverty and the moral value of asceticism to one living in luxury? The counter-question might be: Why not, if that will further the development of the individual and the general welfare?" (Traditional Harmony, vol. 2, 31, 32) In other words, the argument is a bit like that for writing species counterpoint. . . .

In his conclusion, it's obvious that Hindemith is referring to the major dominant ninth, the ø7, and various altered dominants: 

"In respect to harmony and tonality, our pieces will inevitably sound like so many hundreds of the sentimental genre pieces of the post-Tristan style. . . . What was originally felt to be so 'modern' and to promise so much, turns out to be a technical device that is useful only for the creation of all too limited effects. Nevertheless, it is advisable once, for practice, to wallow thoroughly in this style of writing if only to learn through exaggeration what in the end one wants to avoid."

Like Persichetti's harmony, Leon Dallin's Techniques of Twentieth Century Composition was a very influential text in the 1960s and 1970s (edition I looked at: Techniques of Twentieth Century Composition: A Guide to the Material of Modern Music, 3rd ed., 1974). Dallin makes the same point as Hindemith but very explicitly and with prejudice against "popular" music styles:

"Major and minor ninths can be added to all of the seventh chord structures, both diatonic and altered. The dominant ninth was the first to be incorporated into the harmonic vocabulary, but its value to serious composers is impaired by popular connotations and triteness. The sounds of other less hackneyed ninth chord forms are still capable of pungent expressiveness, even to jaded twentieth-century ears, when used with imagination."

Ouch. Still, for the era, Dallin was undoubtedly right. In a later post, I will write that musicians working in the popular styles he denigrates were equally reticent: the statistics will show that the major dominant ninth appears surprisingly infrequently in songs from the 1920s through the 1980s (at least in the songs' published form; performances and arrangements may well differ).

Textbooks, update, part 5 (Persichetti)

 I have added Vincent Persichetti's classic Twentieth Century Harmony: Creative Aspects and Practice to this series of posts, first, because it is in a traditional textbook format and uses a traditional sequence, despite the very different material being discussed, and, second, because it is a good representative (still cited today) of mid-20th century attitudes toward harmony and harmony pedagogy among then-practicing North American and European composers. 

Like other texts that tackled complex harmony beginning already in the early 20th century, Twentieth Century Harmony also stands in the awkward position of prescribing harmonic solutions at a time when harmonic function was quickly losing its hegemonic force. Persichetti's claim isn't small: The book is "a detailed study of the essential harmonic technique of the twentieth century,  presented according to the practice of contemporary composers. This text aims to define this harmonic activity and make it available to the student and young composer."

The magnitude of the problem--and of Persichetti's optimism--is apparent in the opening of chapter 1:

Any tone can succeed any other tone, any tone can sound simultaneously with any other tone or tones, and any group of tones can be followed by any other group of tones, just as any degree of tension or nuance can occur in any medium under any kind of stress or duration. Successful projection will depend upon the contextual and formal conditions that prevail, and upon the skill and the soul of the composer.

It's hard to know what to expect of ninth chords, given those assertions. But under the circumstances, the general statement seems straightforward enough, though it certainly requires the information and examples that follow to be saved from vagueness and to offer any kind of practical instruction:

The seventh and ninth members of chords are traditionally dissonant tones but they have been freed of some of their former restrictions. These chords have become stable entities in themselves with their dissonant tones not necessarily prepared or resolved. Seventh and ninth chords, like triads, may progress within or outside any scale formation, original or traditional. Under certain formal conditions the seventh and ninth are treated as dissonant tones needing resolution; but as independent seventh and ninth chords they have the facility of triads.

A problem common to related literature is the definition of chords. Ninth chords have five members and can be considered complex. As we saw with Sessions, inversions can introduce considerable ambiguity about chordal identity. Persichetti says fourth inversions are used freely, and one example offers an opening chord of A2-G3-F4-B4 as G9. I have trouble hearing this as anything other than Am9, despite the "extraneous" F4. (Hindemith, btw, would agree with Persichetti, as the strongest interval by his calculation is the third G-B.) 

Much the same with non-harmonic notes. Here is the beginning of one example with my annotations:

It is almost impossible to avoid the "reduction to simpler sonorities" priority in cases like these, even if as here those are seventh chords rather than triads.

Finally, after all this, there isn't much to say that connects to the topic of this blog, because--as the example above hints--Persichetti greatly favors other seventh and ninth chord types over the major dominant ninth, which does appear in a reference example of the same kind of sequential progression that we saw in Mitchell and Ottman, but only once in the chapter's nine composed examples.

Textbooks, update, part 4

Ratner

Chapter 11 of Harmony: Structure and Style is on dominant sevenths and the tonic 6/4. Chapter 12 is titled "VII7 and V9--The Major Sixth in Dominant Harmony"; it ends with a helpful summary. Here that is, with further quotes and my comments:

1. The major sixth in dominant harmony appears in two chords, the V9 and the VII7.  [Note that Ratner uses the non-distinctive capital letters of scale-step theory. Since he is writing only about the major key at this point in the book, we know that VII7 is the same as viiø7.]

2. The sixth degree adds a strong characteristic element of color to dominant harmony.  [He is referring mainly to later 18th century music, where the supporting harmony is viiø7: his first two examples are from Mozart and Beethoven. But a brief excerpt from Brahms, Symphony no. 1, II, has a V9 in first inversion--see below--though in typical Brahmsian fashion it sounds as much like an accented neighbor chord as an independent ninth chord.]

 

3. Usually, the sixth degree appears in the uppermost voice, since it possesses marked melodic value.  [In the main text he says ^6 is best in the top voice "where its melodic tendency will be realized in a salient manner."]

4. The sixth will tend to resolve downward to the fifth degree, before the dominant harmony moves to tonic or at the change of harmony. [This "tending to resolve downward" comes from the idea of ^6 as a neighbor to ^5. His options cover my internal and direct resolution categories, respectively.]

5. The sixth may leap downward to another tone of the dominant harmony in a chordal melodic figure, relieving the sixth of the need to be resolved directly.  [His point about this in main text, though, is that ^6 makes a very effective "melodic apex," after which comes a fall to the cadence or other resolution.]

6. In a 6-7-8 pattern the sixth moves upward to the leading tone, and the V9 is first struck without a leading tone.  ["Occasionally, when one voice moves 6-7-8, the harmony momentarily becomes V9 with 6 supplanting the leading tone at first. The musical effect of this can be quite poised and elegant, as shown below."]

7. V° and VII' are usually used within a phrase, except in cases described in summary points 5 and 6.

Sessions

Of all the books surveyed in this series of posts, Session's Harmonic Practice shows the most obvious evidence of an experienced and practically- minded composer. "Frozen" accessory tones are of two types: "Ninth, Eleventh, and Thirteenth Chords" and "Substitute Functions." He offers no method of derivation, just says the ninth chord is "generally classified as a basic chord type," later adds "so-called" to first mention of elevenths and thirteenths, and ends the section with the statement that "in the overwhelming majority of cases, these chords are used on the dominant degree, but use on other degrees is also possible."

The ninth can be resolved internally because the root is already present (he shows both V9 and I9) and thus the ninth can be left by leap internally, but the same cannot be said of the seventh.

As to inversions, they "are extremely varied in harmonic effect, even in the case of different positions of the same inversion, which . . . if they are played in alternation seem to denote a genuine change in harmony." Here is the second inversion of C: V9.

Sessions closes with "For these reasons, it seems better to consider the ninth as an accessory note which habitual usage has 'frozen' onto the chord." Quite sensible from an historical perspective, though he omits to say the same is true of the dominant seventh chord and the add6.

Textbooks, update, part 3

Harder/Steinke

In Harmonic Materials in Tonal Music, Pt. II, 6th ed., the three extended chords are grouped together, and the model of superimposed thirds is assumed. The historical summary I might have written myself: "Used sparingly by earlier composers, ninth, eleventh, and thirteenth chords occur more frequently toward the end of the 19th century. They are especially characteristic of Impressionistic music." The chords may be built on any scale degree, "but the majority are dominant chords."

The chords are definitely regarded as harmonies, but the authors acknowledge that "since these tones often appear as non-harmonic devices, it is sometimes difficult to decide whether they should be interpreted as members of the harmony or as incidental melodic occurrences." Given this quite reasonable statement it seems odd that they do not offer more repertoire examples--there is none with a direct resolution.

Hindemith

The laconic style of Traditional Harmony, vol. 1, was quite deliberate: the volume is really a set of exercises with notes for reference, not a narrative or expository presentation. Hindemith ignores third-stacking except for the dominant ninth chord. He does not mention 11ths, and the 13th originates from substitution; he says "dominant seventh chord with sixth"--which replaces the fifth--but figures the 6 as 13. He allows first and third inversions of the ninth chord, not the second or fourth (implicitly--he just doesn't mention them). Finally, he says that "in progressions, the two derivations [9th and 13th] and their inversions are treated like V7 and its corresponding inversions." The exercises maximize spelling opportunities and as such don't really reflect characteristic practices in existing compositional genres.

Ottman

In Advanced Harmony: Theory and Practice, 4th ed., ch. 10 is divided into three topical units: (1) "chords of the ninth," (2) "eleventh and thirteenth chords," and (3) exercises in "writing ninth chords" plus "the ninth chord in the harmonic sequence."

After an introduction, the sub-sections in the first unit are (1) "ninth chords in which the ninth resolves before a change of root," (2) "ninth chords in which the ninth resolves simultaneously with the chord change," (3) "ninth chords in which the ninth and seventh are arpeggiated," (4) "irregular resolution of the ninth," (5) "ninth chords in sequence." Third stacking is used, and non-dominant ninth chords are accepted but are only "occasionally seen."

Of the ten textbooks discussed in this series, Advanced Harmony has the best arrangement of well-defined sections coordinated with multiple repertoire examples.

Sub-sections 1 and 2 align with my "internal resolution" and "direct resolution" of the major dominant ninth chord. After noting that most internal resolutions can be regarded as melodic figures over V7, Ottman does observe that "When the ninth is held or repeated, a stronger feeling for an independent ninth chord may result. . . . As emphasis on the ninth increases, analysis as a ninth chord becomes more likely, but in any case, the decision is a subjective one at best." This aligns with my emphasis on a melodic-to-harmonic continuum in my "seven types" (link).

Sub-section 3: Here is the reference example with my annotations.

The one example in sub-section 4 is an upward resolution of the ninth, which should be very familiar to readers of this blog and my essays published on Texas ScholarWorks (see the index here: link). Sub-section 5 consists of a reference example showing alternating seventh and ninth chords in a sequential passage--the same progression from Mitchell's Elementary Harmony that I reproduced in the previous post.

Piston/Devoto

In Harmony, 5th ed., chs. 22 & 23 are on seventh chords other than the dominant seventh. Ch. 22 is short, more of a footnote to the preceding: it's on the "incomplete major ninth"--that is, to say the half-diminished seventh or viiø7. The idea that this chord may be added to below--"subtended"--goes back to Rameau and appears in many 19th century texts and treatises. 

Ch. 24, then, takes up the ninth chord proper, which of course is called the "complete dominant ninth."

The author(s) isolate "three important aspects" of the chord:

1. "The ninth may appear as a non-harmonic tone, resolving downward into the fifth degree [my internal resolution], or sometimes, if it is the major ninth, up to the seventh degree [my ascending figure], before the chord itself resolves. It is often an appoggiatura, in which case the harmonic color is very pronounced." 

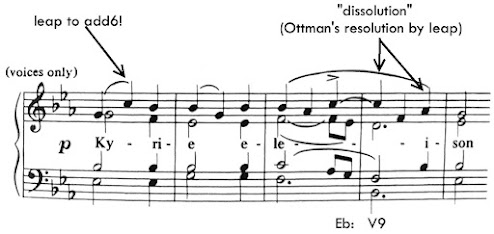
2. "The ninth may be used in a true harmonic sense as a chord tone but it may be absent from the chord at the moment of change. This is an important aspect of harmonic treatment of the ninth in common practice. It actually consists of the resolution by arpeggiation of a dissonant factor, a principle applied to no other dissonant chord (it is also called dissolution by some theorists). . . ." Ottman's 9-to-7 arpeggiation--see the musical example earlier in this post--is one instance of this.

3. "Finally, the ninth may act as a normal dissonant chord tone resolving to a tone of the following chord."  This is my external or direct resolution.

The major dominant ninth has further limitations; it represents a harmonic color characteristic of the end of the common-practice period rather than the eighteenth century. Employed as in the third of the aspects just described, it is rarely encountered until the latter part of the nineteenth century. We will meet the dominant major ninth again in Part Two of this book in connection with impressionistic harmony, in which it is of great importance both as an independent, quasi-consonant sonority and as an adjunct to the triad in modal harmony.

Here is their particularly clear presentation of inversions, with my annotations. The fourth inversion (with ninth in the bass) is rejected.

Textbooks, update, part 2

Forte (none); Laitz (none)

Of the ten textbooks I browsed during a recent visit to a college library, two don't mention ninth chords. These are Allen Forte, Tonal Harmony in Concept and Practice, 2d ed.; Steven Laitz, The Complete Musician, 2d ed. In Forte's case, the reason is clear: his pedagogy is based closely on Schenkerian theory, a conservative (in 2023, one might better say reactionary) model of harmony in which everything is reducible to triads and their linear elaborations. I don't know Laitz's reason--I am relying only on the table of contents and the index, where extended chords including ninths don't appear. It's possible he may say something in the preface or elsewhere.

Gauldin

Ch. 31 of Harmonic Practice in Tonal Music is 11 pages, of which 4 are given to the dominant ninth and just a half page to non-dominant ninths. He does give some space at the end of the chapter to added 6ths and 9ths, which is a positive point.

Gauldin uses a simple "extended tertian harmonies" model. The 9th in the dominant chord, whether major or minor, "became a bona fide member of the V chord" in the later 18th century, which is fair if we mean V(b9) but overstates the case if we mean V9 as an independent chord. The same is true of the claim that "by the middle of the 19th century, composers were using prolonged dominant ninths quite frequently" -- he then quotes the inevitable Franck Violin Sonata (1886) as well as the Prologue to Act I of Götterdämmerung (prod. 1876). European and European-influenced musicians working in all styles were indeed using dominant ninths by this time, but "quite frequently" misrepresents the statistics if by "frequently" one means "commonly" or "often." And "prolonged dominant ninths quite frequently. . ." is simply not the case: "expressive" and "prominent," perhaps, but "not often" if we take the repertoire as a whole before the 1880s. Finally, we note that he doesn't mention inversions.

Early on, Gauldin asserts that "we will regard [the] added thirds as dissonances and treat them as suspension or neighboring figures, much like the chordal 7th in seventh chords." Later examples do have chord labels for V9 but show voice leading figures above. I would read these as direct resolutions: V9 goes to I. Saying that these independent V9s "have their [historical] source in suspension or neighboring figures" would be adequate to clarify the situation.

Mitchell

In Elementary Harmony, 3rd ed., ch. 15 is 30 pages long, the topic being seventh chords, after which 10 pages are given to ninth chords in ch. 16. Eleventh and thirteenth chords are not mentioned. The final chapters are on applied/secondary dominants (17) and modulation (18).

Mitchell distinguishes between "simple" and "manipulated," which correspond to my "internal resolution" and "direct resolution." (For definition and examples, see my early post on the 7 types of the major dominant ninth: link.) Despite the seemingly derogatory "manipulated," Mitchell regards the V9 in direct resolution as a distinct harmonic entity. Later he strengthens the point by calling internal resolutions "pseudo ninth chords." He does, however, return to a "descending natural succession" to describe the origin of the major dominant ninth chord.

He rejects all non-dominant ninth chords out of hand: "they remain details of horizontal motion"--a bit extreme perhaps but still a reasonable description of most music before the 1890s. He says of inversions, "chances are that [they are] the result of simple figuration," but in a later paragraph he rejects them as "fiction" and "alleged." 

Mitchell was also under Schenkerian influence, and we can be thankful that he did recognize the independent ninth chord, even if it still suffers the stigma of being "manipulated."

Ninth chords in harmony textbooks: an update

 Recently I was able to visit a university music library, where I looked at the presentation of ninth chords in the following textbooks. They are not current editions (those are on reserve for students in pedagogy courses), but I have good reason to suspect that these are representative, in other words that little has changed in the pertinent chapters.

Allen Forte, Tonal Harmony in Concept and Practice, 2d ed.
Robert Gauldin, Harmonic Practice in Tonal Music
Paul Harder/Greg Steinke, Harmonic Materials in Tonal Music, Pt. II, 6th ed.
Paul Hindemith, Traditional Harmony, vol. 1
Steven Laitz, The Complete Musician, 2d ed.
William Mitchell, Elementary Harmony, 3rd ed.
Robert Ottman, Advanced Harmony: Theory and Practice, 4th ed.
Walter Piston/Revised and Expanded by Mark Devoto, Harmony, 5th ed.
Leonard Ratner, Harmony: Structure and Style

Roger Sessions, Harmonic Practice

In earlier posts, I wrote about historical textbooks, mostly 19th century, beginning in January 2019: link. The only post-1950 textbook I looked at was the 8th edition of a music score anthology by Benjamin, Horwitz, Koozin, and Nelson: link.

Of the ten books discussed here, five put the ninth chord in the usual, relatively brief chapter late in the volume: Gauldin, ch. 31; Harder/Steinke, ch. 11 (final); Mitchell, ch. 16 out of 18; Ottman, ch. 11 in book 2 of the sequence; Piston/Devoto, ch. 24 out of 31. Forte doesn't mention it at all, not surprising given his Schenkerian loyalties. Laitz doesn't, either. Hindemith, on the other hand, puts the ninth and the thirteenth in chapter 6 (out of 16 total), immediately after inversions of the dominant seventh. Ratner has the same placement, in ch. 12 out of 27, as does Sessions: 9th chords are in §5 of ch. 7 "Accessory Tones"--ch. 6 introduced seventh chords.

Here are repertoire lists for the eight volumes (excluding Forte and Laitz). As a reminder, only items with the major dominant ninth are included.

Gauldin

Kuhlau, Sonatina, op. 20, no. 1, II
Franck, Violin Sonata, I
Wagner, Götterdämmerung, Prologue to Act I
Wagner, Götterdämmerung, Act I

Harder/Steinke

Fauré, "Après un Rêve"
Debussy, Pelléas et Mélisande, Act II, scene 1

Hindemith: only his newly written exercises

Mitchell

Schubert, waltz, op. 50, no. 18
Schubert, waltz, op. 9, no. 34
Beethoven, Quartet, op. 18, no. 6, IV
Schumann, op. 99, no. 11, trio
Schubert, waltz, op. 9, no. 30

Ottman

Verdi, Il Trovatore, Act IV, no. 19
Grieg, In der Heimat
Tchaikovsky, The Nutcracker, Overture
Chopin, Nocturne, op. 72, no. 1
Wagner, Götterdämmerung, Act III
Dvorak, Quartet, op. 105, III

Piston/Devoto

J. S. Bach, WTC II, Fugue in D major
Schubert, Mass no. 6 in Eb, Kyrie
Wagner, Rheingold, scene 2
Haydn, [Piano] Sonata no. 7, II
Chopin, Nocturne, op. 72, no. 1
Mussorgsky, Songs and Dances of Death, no. 3
Franck, Symphony, I
Verdi, Requiem, Requiem
Schumann, Symphony, no. 2, III
Franck, Piano Quintet, I

Ratner

Mozart, Sonata, K. 576, I
Schubert, Fantasia, op. 78
Chopin, Preludes, op. 28, no. 21

Sessions: only reference examples, no repertoire

In the next post, I will begin description and commentary for each book in turn.