Brahms, Waltzes, op. 39, part 2

 In the previous post I showed the direct resolutions of the major dominant ninth (in nos. 2, 7, and 16), plus two others in no. 2. Here are the treatments elsewhere in the set.

The figure 9-8-7, very common in the waltz (especially Ländler) repertoire, occurs three times.

In no. 5 (see second system, second box; figure repeated in the final cadence):

I will discuss the other figures later in this post.

In no. 6 (three times via series of unfolded thirds):

In no. 10: in bar 4 after a scalar ascent from an initial V9; and in the final cadence, where thirds become sixths as 9-8-7 goes into the "right-hand thumb" voice.

A similar figure in no. 7 covers 10-9-8:

Now, to those other figures in no. 7. At (a), the cadence has no dominant ninth. The shape is repeated at (b)--and (b) is repeated, transposed and slightly altered, at (c)--and in these cases there is a ninth forming a major dominant ninth chord. The resolution, however, is ascending: C#5 goes to D#5 in the first instance and F#5 goes to G#5 in the second instance. I don't want to get into the problematic matter of influence, but in this one case I will venture to assert that Brahms borrowed this ascending gesture directly from Schubert. Note, too, that the ascending stepwise line from ^5 to ^8 is repeated in the final cadence against the 9-8-7 figure (see beamed notes in the left hand at (d)).

No. 7 also has an ascending resolution, and it is dramatic, with good metric emphasis to the ninth (see the arrow):

Where my first examples (in Part 1) came from the last waltz, my last examples come from the first, where we find (1) a variant of the (10)-9-8-7 figure in the closing cadence (box at the end), and (2) what I hear as a "just barely" sounding of the ninth in grace notes (boxes in the first and second systems), a characterization that is also justified in that the piece is played without pedal (left-hand staccati preclude it).

Brahms, Waltzes, op. 39, part 1

 The sixteen waltzes of Brahms's op. 39 (1865) manage only six direct resolutions of the dominant ninth chord, four of them in nearly the final bars of the last number. The two earlier examples are both obscured by registral shifts. (Direct resolutions, recall, are external, that is, the ninth over V is resolved in the following I, not before.)

Here is waltz no. 16; the score here lacks only the last phrase and cadence to C# minor.

The voice-leading is impeccable, though I should note that most composers before the 1890s do try to avoid the parallel fifths that can easily arise in resolving the major dominant ninth chord. The invertible counterpoint (cf. bars 1-8 with 9-16) is Brahmsian, not Schubertian!

The resolution in no. 7--see the boxes in the third system--has to be called direct but F#4 goes to E5, not E4, and the contrast is strong: the phrase before is a long descent (starting in the middle of the second system) and the phrase beginning with E5 is an equally strong ascent. I've checked the other versions (piano four-hands and two pianos) and neither has a simple resolution, though it would have been very easy indeed to add the extra notes.

The F#4 is repeated in the left hand (second box) and does move to E4 but the effect is a muddle of A major and D major, made worse if the pedal is held down. The pedal marking, incidentally, is in the manuscript. Note that the composer deleted several before that (arrow).

The other direct resolution is in no. 2, bars 16-17, or from the end of the B section to the beginning of the reprise of A. Here C#3 does go on to B2 but the placement of the ninth very nearly in the bass is unique (at least, I can say that I have never seen it elsewhere, not even in the more adventurous musics of the 1890s and early 1900s) and of course it is completely contrary to all advice about how to treat the major dominant ninth chord (recall that even inversions are suspect).

Two other points of interest in this score: (1) In bars 4-5, what I call an "almost direct resolution", where unfolded thirds clearly show the voice-leading as C#6-B5 and A5-G#5; (2) an expressive highpoint in the final cadence--a cliché of the waltz going all the way back to Schubert's generation--and an internal resolution, if one regards the entire bar as V and, even better, holds the pedal down (which I have never done; the "clean" sound of the higher register ii6 is more appealing).

In a subsequent post I will provide examples of the other internal resolutions and evasions.

Brahms, Symphony no. 2, III

The Allegretto quasi Andantino sits in the position of a 19th century sonata's dance movement. Its ABABA design is in the tradition of the Beethoven symphonic scherzi, including the sharp contrast between sections: A is a pastorale/menuet(?), and B is an agitato/galop(?). Brahms also hints at a Schubertian Ländler with the leap to and from ^6 in bar 2:

Note, however, that the harmony is viiø7, not V9. In fact, the piano reduction has left out a note: a continuing G3 in the bassoons, of course confirming the pastorale topic.

There is a proper V9 in bar 22 (boxed in the first graphic above), but no simple resolution of the 9. 

The pattern continues in the variation of the theme at the beginning of the B-section and in the first reprise of A.

The same is true of the F#-major reprise (circled), but leading into it is a very expressive V9 (boxed), where the 9 is resolved internally in a gesture one finds in Schubert waltzes and also in Brahms's hommage to them, op. 39.

In the ending, another brief moment of a root-position V9 (circled) is undermined by a chromatic descent and ascent. And a big invitation to what was by the 1870s a cadential cliché--V9/V--lacks its bass and so is another viiº7 (boxed). Brahms sets up the conditions so well that one wonders if the bass players thought they had been given the wrong note.

We'll find the situation somewhat different in the Waltzes, op. 39, but in general Brahms was more conservative than his best-known contemporaries in his treatment of the major dominant ninth and the upper tetrachord of the major key.

Harmony at the Ninth: The repertoire problem

 In a previous post, I outlined a harmony pedagogy that would place the ninth chord near the beginning of the curriculum, not—as is typically the case (if it's taught at all)—somewhere near the end. I also noted that my plan got into trouble at the point of choosing repertoire. I'll discuss the three main reasons here: (1) repertoire bias in the traditional theory core curriculum; (2) conflict between 18th/19th century and 20th/21st century theoretical models; (3) difficulty in finding entirely diatonic examples suitable for first-year theory classroom use.

To begin, I have assembled and posted to my Google Drive a list of all the musical examples for the five essays on the dominant ninth chord that I have published on the Texas ScholarWorks platform to date: 

V9 essays repertoire list. 

The essays are named at the beginning of the file, and abstracts and links are provided.

(1) repertoire bias in the traditional theory core curriculum

The historical narrative for classical music that prevailed through much of the 20th century was progressive, that is, it began from one point (usually medieval chant) and led by more or less regular steps forward into contemporary music. So, we have the first inklings of counterpoint around 1000 AD, eventually a perfected polyphony in the 16th century, an organized major/minor tonal system thanks to continuo practice and pedagogy in the later 17th and early 18th centuries, and a gradual expansion or break-down of that system through more complex harmonic relations and increased chromaticism in the 19th century, till we reached a fully chromatic model epitomized by twelve-tone and serial music. Despite this scheme, the heart of the story remained with the High Classical period (sometimes called the First Viennese School) with Haydn, Mozart, and Beethoven.

This is the narrative I learned as a young student. We had only just begun to acknowledge some of its problems even by the time I started college in 1968. For my purposes here, though, and beyond noting that these repertoire biases have barely changed in mainstream college introductory theory textbooks, the one point that is immediately relevant can be easily understood by a quick comparison of the repertoire list linked above with two textbook-based lists. The smaller of the two is derived from Kostka & Payne, 3rd ed. (even earlier than my 4th ed. copy!): David Temperley corpus study: see the bottom of that web page. The larger is the table of contents for the score anthology by Benjamin, Horvit, Koozin, and Nelson, Music for Analysis, 8th edition (2018).

Temperley extracts the 46 longest examples from the Kostka & Payne workbook. Of these, 29 are by Haydn, Mozart, Beethoven, and Schubert. For these 29, 11 are from piano sonatas, 3 from other pieces for piano, 9 from chamber music, 2 from concertos, 3 songs, and 1 opera.

Benjamin, Horvit, Koozin, and Nelson has 477 examples ranging from the 17th century to the present. Of these, 378 are prior to 1900, with 175 by Haydn, Mozart, Beethoven, and Schubert. For the 378, 49 are from piano sonatas, 12 from other pieces for piano, 32 from chamber music, 2 from concertos, 15 songs and vocal ensemble music, and 5 operas. In addition, 24 are dances in keyboard format, and 37 are from orchestral ensemble music (symphonies and overtures).

For reference, in Benjamin, Horvit, Koozin, and Nelson there are two examples from Johann Strauss, jr., while in Temperley's extracts from Kostka & Payne there are none—which points up the problem: there is very little intersection between their lists and mine, in which Johann Strauss, jr. and sr. dominate. Some important caveats: Apart from the Strausses, my study of the major dominant ninth chord is skewed toward the decades surrounding 1900. As I have noted in essays, I have generally looked at shorter compositions; for longer works, I use keyboard reductions rather than full scores but I haven't focused on large instrumental ensemble music and have done even less with chamber music. I have studied dances—especially polkas and waltzes—throughout the 19th century, not just Schubert dances. The historical circumstance that ascending cadence gestures, upper-register cadences, and clear treatment of the major dominant ninth all seem to have arisen in music for dance led me to the larger repertoires that incorporated them, beginning with opéra comique in the 1830s, then blossoming in operetta in the 1850s and later. In general, composers—including Schubert himself—would be more conservative when writing in the larger instrumental forms than in the popular forms of dance music and music for the stage. In another post I will report on my look at the Allegretto grazioso quasi Andantino in Brahms's Symphony no. 2 and at his Waltzes, op. 39. Even in the midst of the Schubert craze of the 1860s—to which he also contributed—Brahms was a genius at suggesting but avoiding the two characteristic chords of scale degree ^6: the dominant ninth and the add6.

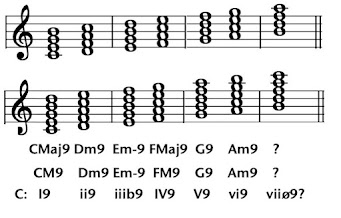
(2) conflict between 18th/19th century and 20th century theoretical models

Textbooks still lump the “extended chords” together, in a model of progressive stacked thirds, even if, as Kostka & Payne remark in their 4th ed.: "Just as superimposed 3rds produce triads and seventh chords, continuation of that process yields ninth, eleventh, and thirteenth chords (which is not to say that this is the manner in which these sonorities evolved historically)." (!!) Jazz theory, on the other hand, doesn't bother with that, because the harmonic vocabulary is based on the dominant seventh with a variety of sounds, "tensions," and alterations built on it. In Mark Levine's Jazz Theory Book (1995), for example, "extensions" (9th, 11th, 13th) are listed in the glossary, but the ninth chord is never explicitly introduced in the text. Instead, it simply appears in the first example for the II-V-I progression:

This version of the opening of "Stella by Starlight" is a concise catalogue of the three main 9th chord types, but note that none of Levine's chord labels (above the score; mine are below in blue) indicates a 9.

Here are some additional examples drawn from different places in The Jazz Theory Book. My labels are below the score. The 9 is included in the fourth chord only because it is altered (G-nat = Fx).

(* I am grateful to UT-Austin doctoral alum and friend Joel Love for telling me about Levine's book. Link: His web page.)

(3) difficulty in finding entirely diatonic examples suitable for first-year theory classroom use

My first examples would, of course, come from Schubert waltzes, but it turned out to be difficult to find simple examples of V9 without also including chromatic chords. Here is the second strain of Valses nobles, D. 969, n11 (1828), with its "textbook perfect" V9 with a direct resolution. Bars 5-6 would require some discussion, however.

In their section on the dominant ninth, Benjamin, Horvit, Koozin, and Nelson include Strauss's Künstlerleben, one of the best known of his mid-period waltzes. I don't know what they say about it, as I don't have a copy of the anthology, but I can say that I find the choice of no. 3 particularly good because the ninth appears several times in different roles, and the only chromatic consideration is a relatively simple cadence to V at the halfway point. At (a) and (c) are internal resolutions (9 resolves within V). At (b) and (d) are "almost direct" resolutions (9 is held over the first part of I); the example below the score shows what a direct resolution would have been in (b). At (e) is the upward resolution of 9 that facilitates an ascending cadence. And at (f1) & (f2) is a very common device that flips the functional status of scale degrees ^7 & ^6: at (f1) ^7 is a simple chord tone and ^6 forms the ninth, but at (f2) ^7 is an appoggiatura and ^6 is a simple chord tone.

Simplified, correct, but not as expressive version of bars 6-8:

The question of repertoire choices appropriate for different levels can be explored through the repertoire list mentioned and linked to at the top of this post. I can add here that I have studied but have not yet reported on stage works from opéras comiques of the 1830s (mainly Adam, Auber) to operetta (Offenbach, Lecocq, Strauss), Savoy opera (Sullivan), and American operetta (Herbert) and musical (Kern). And of course there is something still to be said about that "genius of avoidance," Brahms.

Harmony at the Ninth

In the past year or two, a movement toward curriculum change in college-level music theory ("reform" is the word more often used) has rapidly accelerated. Research had already expanded notably into new repertoires, especially music of all styles in the late 20th and 21st centuries. The journal demonstrating this most strongly is Music Theory Online (link), a venerable peer-reviewed journal of the Society for Music Theory, and one can also see the effects on teaching in recent volumes of the Journal of Music Theory Pedagogy (link). Other major journals are beginning to follow their lead.

Reading recently about one group's proposal to remove or severely limit the use of chorale harmonizations (almost always those by J. S. Bach), I decided to experiment with another change. What if large-market music theory textbooks took the dominant ninth chord out of its late-chapter ghetto and put it at or near the beginning of the book instead? 

I'll use the 8th edition of Kostka, Payne, and Almén, Tonal Harmony with an Introduction to Post-Tonal Music (2017; publisher link) as my representative example. The book has 28 chapters. The topics covered are: in the first four, fundamentals; in the next eleven, diatonic triads and seventh chords; in the following ten, chromaticism; and in the final three, post-tonal theory. The second section on chromaticism encompasses chapters 21-25: 21 & 22 introduce the usual Neapolitan chord and augmented sixths, which are already found in 17th century sources and become staples of expressive harmonic practices in the 18th century; 23 is on enharmonic spellings; 24 is titled "further elements of the harmonic vocabulary"; and 25 carries us through the late 19th century.

I am now switching editions because the publisher's link for the 8th edition, which I do not own, does not show chapter subheadings. Some time ago I purchased a copy of the 4th edition (2004) for $1.00 at our local Half-Price Books Outlet store. A comparison of the tables of contents for the 4th and 8th editions suggests little has changed overall. The "further elements" chapter has five sections, the first two being on altered dominants. In the third section we finally reach "ninth, eleventh, and thirteenth chords." There are five musical examples, two from Beethoven, two from Schumann, and one the inevitable Franck Violin Sonata opening. Only the latter shows the major dominant ninth; three are Vb9, and one is ii9.

From less than four pages at the back of the book to a place of honor at or near the beginning of the curriculum would seem an impossible task, but actually I found it easy--but only up to a point. The difficulty was repertoire examples. I'll report on that in a subsequent post.

To begin: I assume that the student has covered fundamentals, including clefs (at least treble and bass), note names, intervals, scales (at least major and minor), and spelling triads and seventh chords. Knowing something about Roman numeral chord labels and jazz/popular chord symbols would be helpful, though they could be taught in conjunction with the text here.

Here are the four types of triads: major, minor, diminished, and augmented. The first three can be found in the major scale. The first line of labels is from jazz/popular-music theory; since labels can vary quite a bit, I chose to use the ones on the Berklee College of Music page Glossary of Terms (link) under “Terms Used in Harmony [1-4].” The second line has labels associated with guitar chord symbols.

The third line has Roman numeral labels, the type most commonly used in traditional undergraduate harmony textbooks. An alternate version you’ll sometimes see doesn’t have distinctions of “chord quality” (that is, major, minor, diminished, augmented) but just capital letters, so for the first three in the graphic I, II, VII. This system has trouble with accidentals so there’s no easy label for the last one, and in general this “all-caps” scheme is less useful than the others, and worse, although it does simplify things, it forces one into accepting a model where everything else is a variant of the scale-based triad.

Seventh chords add a scale note a third above the triad. The major triads produce two very different types. The first one  is the “major-minor seventh chord” (major triad plus a minor third) or “dominant seventh” or “V7” or just “seventh chord” or “7”—in most music of the last century when you say “seventh chord” this one is the default. Notice that the label in the graphic has no qualifier—it’s just “G7.”

The second chord in the graphic is the other one built on the major triad: the “major-major seventh chord” or “major seventh chord.” It requires a qualifier “Maj” or “M” except in Roman numerals, which, oddly, distinguish quality of triads but not seventh chords; in the graphic below, V7 and I7 look the same, but they’re not.

The V7 chord is unique in the major scale; the major seventh chord is not. The graphic below shows all seven triads in the C major scale aligned with the seven seventh chords. 

Here, G7 is on its own, but there is both CMaj7 and FMaj7. Notice that there are actually three minor seventh chords: Dm7, Em7, and Am7. The last one in the graphic is again unique, but as we’ll see later it’s very closely associated historically with the dominant seventh chord and the dominant ninth chord. The most common jazz/popular music label is pretty clumsy: read it as “B minor 7 flat 5.” Not that the others are much better: read Bø7 as “B half-diminished 7” and viiø7 as “seven half-diminished seven.” Why “half-diminished” would get us off on a tangent, but I don’t want to ignore the chord completely because of its connection to the dominant ninth. On a Mac keyboard, by the way, you get ø by typing option-o.

In the harmonic series, that wonderful source of symmetries and patterns in musical sounds, the dominant ninth shows up immediately after the dominant seventh. In the graphic below, first there is the G major triad (nos. 1-6), then the seventh (at position no. 7), then the ninth (at position no. 9; see the arrow!). That’s the explanation for this essay’s title, by the way: Harmony at the ninth. The red brackets show two ways to read the dominant ninth chord with consecutive tones of the harmonic series. The lower bracket hints at the traditional derivation of ninth chords by adding a third above the seventh.

[Note 3-24-2022: I forgot that this derivation is an old one, going back to the first years of the Paris Conservatoire. For more, see my blog post on Charles-Simon Catel's Harmonie: link.]

One of the graphics above showed triads and seventh chords aligned. Here is the analogous one for seventh and ninth chords. 

This looks daunting but there really are just a couple changes of any importance. The chords on C and F are still the same: Cmaj9 and Fmaj9. So are the chords on D and A: Dm9 and Am9. On the other hand, the chords on E and B have collapsed into things that are unusable—which is to say that musicians in the later 19th and early 20th centuries did their best to avoid them (or they would alter notes in them). 

The chord on G is special. It succeeds in combining a similar characteristic of G7 and Bø7, specifically, the expressive dissonance at the top of G7—the note F5 in the graphic—is added onto and intensified by another expressive dissonance at the top of G9 (the note A5). Nevertheless, the seventh and ninth are different: go back to the harmonic series above The notes at positions number 7 & 11 (marked with an asterisk) are both “out of tune,” that is, difficult to bring into harmony with other pitches in scales or intervals in any familiar tuning system. People have known this for a long time, hence, the medieval diabolus in musica (“the Devil in music”), which would apply to the tritone B4-F5 here. But notice that the ninth A5 does not sit at the top of a tritone; it belongs to the perfect fifth D5-A5 and thus renders an arguably more consonant (or well maybe less dissonant) sound in the dominant ninth chord compared to the dominant seventh chord. Musicians in the 19th century recognized this and made the most of it.

We’ve reached the dominant ninth through chordal derivations so far, but there is another way to look at it, through the major scale. In this graphic, a C major scale appears at the left, my parsing of it at the right. Of the seven pitches, the dominant ninth (above) uses up five of them, whereas the C major triad (below) has only three. The practical implication of this—not lost on 20th century musicians especially—is that the dominant ninth can easily be used to harmonize quite a few melody tones.

In the common sequence of topics, this is where I would either keep on going and introduce the last two of the so-called “extended chords”—the eleventh and thirteenth chords—or I would discuss voice-leading for the dominant ninth chord. Although I acknowledge, just like I did with the ninth chords, that stacking up the thirds makes these chords easy to spell and remember, the problem is that the “extended chord” scheme is completely wrong historically. First of all, the eleventh and thirteenth chords both derive from substitution, not from adding more thirds: the eleventh takes the place of the tenth and the thirteenth takes the place of the twelfth. Second, one almost never sees these chords in their full form with six or seven notes: two or even three notes are commonly omitted. Discussing them now, in other words, would take us far afield from our main topic, which is how we can learn some essentials of tonal harmony by starting from the dominant ninth chord.

As to voice-leading, those discussions quickly collapse back into giving priority to 17th and 18th century rules (which, to be sure, are not entirely arbitrary but are based on 17th and 18th century practices). Voice-leading doesn’t work quite the same way after about 1860 (or thereabouts), and it was the dominant ninth that led the way, fueling a different attitude toward the upper part of the major scale, or scale degrees ^5-^8, G-A-B-C in C major, and even for the prohibition on parallel fifths. 

I have written about these historical issues elsewhere in this blog and in essays published on Texas ScholarWorks: see especially "The Dominant Ninth in Music from 1900 to 1924, Part 1" and "The Dominant Ninth and Tonic Seventh in the Upper Tetrachord of the Major Key."

More on the repertoire problem in a subsequent post.